Research Article, J Nucl Ene Sci Power Generat Technol Vol: 12 Issue: 1

Highlighting of the Local Neutronic Efficiency Distribution in the MEGAPIE Spallation Target by GEANT4 Modeling

Abdesslam Lamrabet^*, Abdelmajid Maghnouj and Abdessamad Didi

Department of Physics, University Sidi Mohamed Ben Abdellah, Morocco

*Corresponding Author: Abdesslam Lamrabet
Department of Physics, University Sidi Mohamed Ben Abdellah, Morocco
E-mail: abdesslam.lamrabet@usmba.ac.ma

Received date: 05 September, 2022, Manuscript No. JNPGT-22-73670; Editor assigned date: 08 September, 2022, Pre QC No. JNPGT-22-73670 (PQ); Reviewed date: 22 September, 2022, QC No. JNPGT-22-73670; Revised date: 03 January, 2023, Manuscript No. JNPGT-22-73670 (R); Published date: 16 January, 2023, DOI: 10.4172/2325-9809.1000317.

Citation: Lamrabet A, Maghnouj A, Didi A (2023) Highlighting of the Local Neutronic Efficiency Distribution in the MEGAPIE Spallation Target by GEANT4 Modeling. J Nucl Ene Sci Power Generat Technol 12:1.

Abstract

Keywords: Neutron production; Secondary protons; MEGAPIE target; GEANT4; Spallation reaction

Introduction

The MEGAPIE project has demonstrated the feasibility of a PbBi liquid metal spallation neutron source able to power an Accelerator Driven System (ADS) [1-3]. Such a source is a coupling of a PbBi spallation target with a particle accelerator to maintain criticality in the sub-critical systems (ADS). The feasibility has been well demonstration, both theoretically and experimentally, during the period 2000-2014. Neutron studies conducted before and after the experimental demonstration were able to characterize the neutronic behavior and performance of the MEGAPIE target [4,5]. However, this characterization remains quite global and is not sufficient to undertake an easy and efficient optimization for this target. That is why there are almost no optimization studies of this target in the scientific literature. Currently, the MEGAPIE jargon uses the spallation zone to refer to the active or neutron producing area of the target and almost never talks about the effective spallation zone and especially the spallation sites. In fact, the identification of these centers is essential to make significant improvements to the target through limited and localized material and/or geometrical modifications. In this paper, we propose to evaluate the local neutron performance of the MEGAPIE target in order to highlight new information to deepen the understanding of its neutron behavior and thus make its optimization more accessible and efficient. Here, we are talking, in particular, about the distribution of the locations of the highest neutronic productivity, namely the most efficient spallation sites. For this purpose, we have proceeded with the following calculations: As a first step, we have evaluated the axial evolution of the average rate of produced neutrons on cumulative volumes along the z-axis of the target. Then, we have explored the local distribution of this rate in the whole active zone. Finally, to confirm some conclusions, we have also calculated the local rate of secondary protons. Unlike most neutronic studies of the MEGAPIE target which are mainly carried out with the MCNPX and FLUKA codes, the present study is performed using the modern Monte Carlo code GEANT4 [6-12]. It is based on the new object oriented C++ programming technology and having a toolkit character. This means that for each simulation task, GEANT4 offers the user a wide variety of choices to satisfy different modeling needs.

Materials and Methods

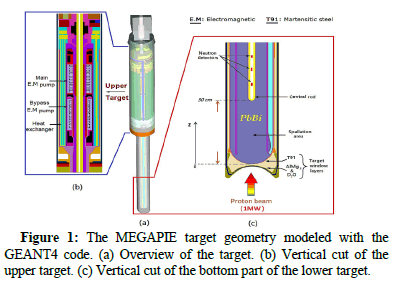
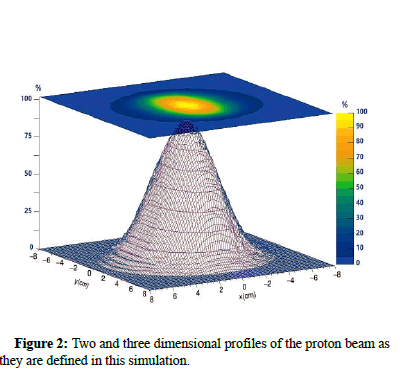
The reliability of this code to reproduce the valid results of the MEGAPIE target has already been demonstrated in our works [13,14]. In the present modeling, we have defined the target geometry (Figure 1) and the profile of the primary proton beam in the same way as in the two previous references. The principal characteristics of the proton beam are: Mono-energetic of 575 MeV of energy and 1.74 mA of intensity. One dimensional according to the target’s axis and having a planar spatial distribution with a quasi double Gaussian profile (Figure 2). The main modifications made to this simulation compared to the previous ones are the use of parallel geometry (§ 2.2) and convenient scorers to extract useful data. The study led to the following results: The spatial distribution of the average and local neutron production, the local secondary proton production, the determination of the spallation reaction centers and the distinction between the spallation region and the neutron production one. All results are consistent and lead to the same conclusions.

Modeling code

GEANT4 (Geometry and Tracking 4) is a simulation environment for tracking particles in matter. The GEANT4 tool was launched in 1994 as part of the RD44 project in order to overcome the encumbrance of its precursor "GEANT3". Unlike GEANT3 which had been written in Fortran, GEANT4 has benefited from the new C+ +/OO programming technology which, thanks to its design marked by independence between its components, has made it flexible and easy to be improved and maintained. The GEANT4 initiative was undertaken by the European Organization for Nuclear Research (CERN) and the Japanese high energy accelerator research organization (KEK). The first version GEANT4.1.0 was released in December 1998. The toolkit character of GEANT4 allows the user to completely build and customize his own application without being limited to predefined components of the code. Indeed, the Object Oriented Programming (OOP) technology allows users to modify the available implementations and/or add new ones without affecting the basic architecture of the code. GEANT4's capabilities have, in fact, made it a platform for building other more specific codes, including codes dedicated to medical applications such as GATE [15-17] and GAMOS and the MCADS model [18-23] for modeling spallation targets. In this work we have used the GEANT4.10.2.p03 version built on a scientific Linux v7.4 system together with many other components to insure a better performance. The required components are: The class library for high-energy physics CLHEP v2.3.1.1], the data analysis framework ROOT v6 and the development framework Qt v5.6.2 [24-28].

The parallel geometry feature

Modeling real experiments, such as the MEGAPIE target, often involves devices with complicated geometry and material composition. This complexity is more apparent in some modeling tasks than in others. Among those affected by this complexity, we point to the extraction of useful data, the definition of user limits, the configuration of variance reduction, etc. This is particularly the case when a modeling operation involves several components at the same time. To circumvent the problem and overcome the complexity, GEANT4 offers two types of geometry: Mass geometry and parallel geometry [29]. The mass geometry is the real geometry of the simulated device equipped with the real materials. The mass geometry of the MEGAPIE target. The parallel geometry is a fictitious geometry to which no materials are assigned. This geometry is defined in the same way as the mass geometry. It has the particularity of being free of the restrictions related to volume boundaries. In other words, overlapping and sharing of surfaces are not prohibited. The only restriction is that it should not extend beyond the global volume. In terms of use, a volume in parallel geometry is just like any other volume in mass geometry, it can serve as: Sensitive detector for scoring purposes. Region to define user limits. e.g., maximum step, range cuts, minimum energy to track a particle. Region to define the biasing. e.g., assigning an artificial weight.

In the present modeling, we have used parallel geometry for two main purposes: Implementation of the geometric importance based variance reduction and definition of sensitive detectors that cover multiple volumes in the mass geometry. It should be noted here that in the MEGAPIE target, data extraction becomes so delicate if parallel geometry is not used. Indeed, to extract a given physical quantity, we must associate to each concerned volume of the mass geometry a distinct sensitive detector. Whereas with parallel geometry, a single volume can gather all juxtaposed volumes regardless of their material composition.

Modeling physics

The physics used in this simulation is designated in GEANT4 by the nickname "FTFP_INCLXX_HP". This is a predefined physics list provided by the code and known as the most appropriate physics list for spallation problems [30]. It includes the following physical models: the Fritiof model (FTF) [31,32], the Pre-compound model (P) [33], the C++ version of the Liege Intranuclear Cascade model, (INCLXX) and the High Precision model (HP). to be more adapted to the studied problem we coupled on the one hand, the INCLXX model with the ABLA [34] statistical model dedicated to the de-excitation phase of the spallation reaction and on the other hand, the HP model with the thermal neutron scattering model "G4 Neutron HP Thermal Scattering" dedicated to the neutron processes in the energy range (˂4eV).

For more details about the cited models, including the types of simulated particles, the energy ranges, the way each of them is used, we refer the reader to our work, where we have described the same physics more explicitly.

Results and Discussion

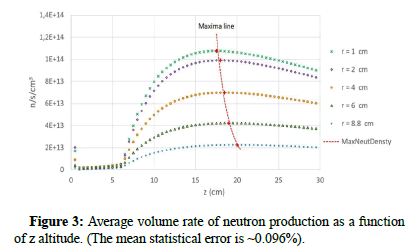
One of the main keys for an easy and successful optimization of the MEGAPIE target is to have detailed information on its neutron performance. But so far, most of the available quantities, such as: Fluxes, flux spectra, number of neutrons by primary proton, etc. are all global quantities or correspond to particular positions in the target. Such quantities do not allow easy consideration of efficient material and/or geometric modifications to improve the current design. For this reason, we have evaluated other physical quantities that show how the productive potential of the target is distributed throughout the entire active zone, which allows for a deeper understanding of the neutron behavior of the target. The quantities we are talking about are simply the local yield of neutrons and secondary protons. Both quantities are calculated by volume unite of (1 cm³) in different positions of the production area. As a first step, we have assessed the axial evolution of the average volume rate of produced neutrons by time unite for different radii. The volumes considered are cylinders centered on the target axis and having different altitudes z all start from the target window (z=0 cm). Sampling was performed along the z-axis with a step size of △z=0.5 cm up to a limit of (z=30 cm). Figure 3 shows the evolution curves of the neutron rate in these volumes. Analyzing these curves, we see that after a small fluctuation near the window (0 ≤ z ≤ 0.5 cm), the volume rate of neutron production remains low and roughly constant until the level of z=~6 cm where it suddenly undergoes a strong increase until it reaches its maximum. After that, it slightly decreases or remains roughly constant when the radius becomes large enough. In addition, the line of maxima in Figure 3 shows that the larger the radius of the volume studied, the greater the z-altitude required having a maximum neutron rate. Indeed, for a radius of r=1 cm, the average of neutronic productivity reaches its maximum at z=~17 cm, while for (r=8.8 cm) that represent the target’s radius, the maximum is marked at z=~20 cm.

The interpretation of these findings has allowed us to conclude that specific reactions, in terms of neutron production, start to occur in the target as soon as the altitude z=6 cm. The reactions that could be responsible for the changes in neutronic productivity observed above are generally spallation and/or multiplication reactions (n, xn). But considering the following semi empirical formula λmfp ∝ A^1⁄3 [42], which gives the relation between the mean free path λmfp of the spallation reaction induced by protons in a heavy metal and the mass number A of this metal, we can conclude that the relevant reaction is most probably spallation. Indeed, the liquid metal PbBi, which represents the actual spallation target in the MEGAPIE target, is mainly composed of lead (Pb, A=206, ~45%) and bismuth (Bi, A=209, ~55%) and the spallation mean free path of such a composition is actually, according to the previous formula, about λmfp ≈ 6 cm. However, spallation reactions cannot occur continuously in all the active zone of target, but mainly in regions separated by distances around λmfp. This shows that the neutron production between two consecutive generations of spallation reactions is mainly ensured by other reactions as (p, xn), (n, xn) and eventually (n, f) fission reactions.

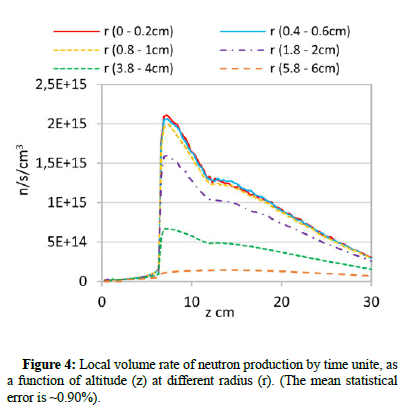
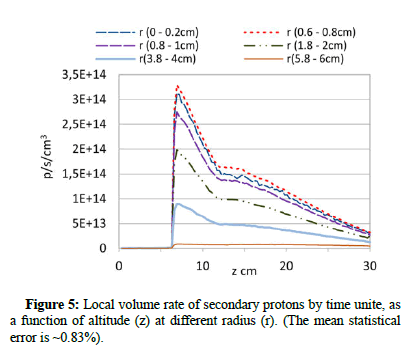
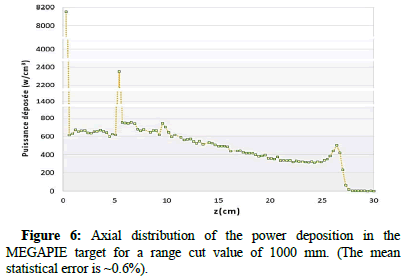
The above results reveal that spallation reactions occur remarkably only after about 6 cm altitude, but how their positions are distributed in the active zone? Is still not revealed. To answer this question, we proceeded to a local evaluation of the same neutronic quantity. In contrast to previous calculations, which were based on cumulative volumes, here we have estimated the neutron production rate on very small separate volumes. We have divided the most neutron contributing area (0 ≤ z ≤ 30 cm and 0 ≤ r ≤ 6 cm) into small cylindrical strips centered on the target axis, each having 3 mm height and 2 mm radial thickness. The obtained results are represented in Figure 4. The most notable observations in this figure are the sharp peaks at about z=7 cm and the nearly horizontal portions in ~12 cm ≤ z ≤ ~14 cm. In the remaining interval of z altitude, the neutron production rate only undergoes a steady decrease. In fact, these observations are only quite clear in the r ≤ 4 cm radius range. For higher radius, the peaks disappear progressively and the production rate becomes almost invariant with altitude. The fact that in the position of the peaks, the neutron production rate suddenly increases by a factor that can reach 20 or even more, proves that this position is a real center of spallation reactions. This is because only the spallation reaction that can reach this multiplication factor. Since the spallation reaction has the particularity of producing, at the same time, a large number of neutrons and protons and to verify the previous conclusions, we have also calculated the local rate of secondary protons. The obtained results are illustrated in Figure 5. They seem perfectly similar to those of the neutron production rate and show the same singularities, which confirms the conclusions made above. Finally, invoking the power deposition from the results obtained in our work [13], we can definitely confirm that two main generations of spallation occur in the active area of the MEGAPIE target: The first at the region ~6 cm ≤ z ≤ ~7 cm and the second at the region ~12 cm ≤ z ≤ ~14 cm. Figure 6 shows especially four singularities.

The first, results from the first contact of the energetic proton beam with the target. The latest one is the Bragg peak. The two other singularities are due to spallation reactions as it has been explained. It is worth noting here that the results of were obtained with a specific setting of the GEANT4 simulation. In particular, we refer to an intrinsic parameter of GEANT4, called "secondary production threshold or range cut". This parameter is set to an unusual value (1000 mm) in order to study its impact on the power deposition in the MEGAPIE target. The definition and the impact of the range cut on the power deposition in the MEGAPIE target is detailed in our paper.

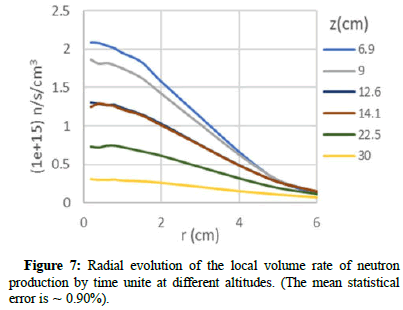
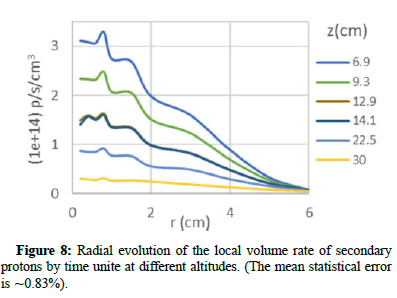
To complete our view of the neutronic performance distribution in the active zone of the MEGAPIE target, let us now examine the radial evolution of the local neutron and proton production rate. Figure 7 and 8 illustrate the evolution of these two quantities respectively. They show that the productivity in neutrons and protons decreases rather quickly from the center to the surface and from about r=~6 cm, this productivity becomes quasi uniform. But contrary to the smooth decrease of neutron production, the production of secondary protons shows some kind of repeated bumps. This means that the concerned positions are places of a certain type of (X, xp) reactions. The abundance of neutrons at the center of the target, leads us to think of (n, p) reactions, while keeping in mind the possibility of electromagnetic reactions such as photo-nuclear reactions (γ, p). But whatever the type of interaction, the observed undulations show that the incident particles that induce the proton production reactions reach the positions concerned with energies that favor the reaction of a given isotope, that is, the one that presents a large effective cross section with respect to this reaction. Nevertheless, the observed undulations phenomenon still requires further studies to be fully explained.

Conclusion

The present work was devoted to the search for new information that could be the basis of any optimization approach of the current MEGAPIE target design. The calculations are performed using the Monte Carlo method based on the GEANT4 toolkit. By evaluating the local productivity of the target in neutrons and protons, we were able to redefine the spallation zone and to locate these occurring centers. The obtained results affirm that the area usually called "spallation region" contains in fact only two main spallation layers that strongly contribute in neutron production of the MEGAPIE target. These layers have a quasi spherical shape and are arranged horizontally in the following two respective regions: ~6 cm ≤ z ≤ ~7 cm and ~12 cm ≤ z ≤ ~14 cm. Perhaps there is a third or more generation, but certainly their contribution to neutron production is indistinguishable from other reaction types such as (n, xn) and (n, f). Thus, the so called spallation region 0 ≤ z ≤ ~30 cm is in fact, the region of general production and not of spallation one. The true spallation region is practically extended from z=~6 cm to z=~14 cm relative to the target window. Moreover, the calculations revealed a particular radial behavior concerning the secondary proton production. But the interpretation of this behavior led us to conclude that further studies are needed to explain it completely.

Finally, the results of this study together with those of our previous study can indeed help to optimize the MEGAPIE target by bringing much focused material and/or geometrical modifications. The issue is that the target becomes more efficient, either by producing more neutrons with the current primary beam or by keeping the same production with a lower intensity beam, thus preserving the target structures from irradiation damage and extending its lifetime.

Acknowledgments

All calculations are performed on the high performance cluster “HPC-MARWAN” of the National Center for Scientific and Technical Research (CNRST) in Morocco. The authors would like to thank all the staff of HPCMARWAN.

References

1. Salvatores M, Bauer GS, Heusener G (2000) The MEGAPIE Initiative. PSI Bericht Nr 01-05.

   [Google Scholar]

2. Latge C, Groeschel F (2006) Megapie spallation target: Design, implementation and preliminary tests of the first prototypical spallation target for future ADS. Actinide Fission Product Partitioning Transmutation Ninth Information Exchange Meeting, Nimes, France. 407-419.

   [Google Scholar]

3. Bauer GS, Salvatores M, Heusener G (2001) MEGAPIE a 1 MW pilot experiment for a liquid metal spallation target. J Nucl Mater 296:17.

   [Crossref] [Google Scholar]

4. Zanini L, Aebersold HU, Berg K (2008) Neutronic and nuclear post-test analysis of MEGAPIE. PSI Bericht Nr 08-04.

   [Google Scholar]

5. Michel-Sendis F, Chabod S, Letourneau A (2010) Neutronic performance of the MEGAPIE spallation target under high power proton beam. Nucl Instrum Methods Phys Res Sect B 268:2257-2271.

   [Crossref] [Google Scholar]

6. Pelowitz DB (2005) “MCNPX User’s Manual Version 2.5.0”. Los Alamos National Laboratory report.

   [Google Scholar]

7.  McKinney GW, Durkee JW, Hendricks JS, James MR, Pelowitz DB, et al. (2005) MCNPX 2.5.0-New features demonstrated, American nuclear society, LaGrange Park, IL, LA-UR-04-8695.

   [Google Scholar]

8. Fasso A (2000) in FLUKA: Status and prospective for hadronic applications. Proceedings of the Monte Carlo 955-960.

   [Crossref] [Google Scholar] [PubMed]

9. Fasso A, Ferrari A, Sala PR (2001) Electron-photon transport in FLUKA: Status in advanced monte carlo for radiation physics, particle transport simulation and applications. Springer 159-164.

   [Google Scholar]

10.  Agostinelli S (2003) GEANT4-a simulation toolkit. Nucl Instrum Meth A 506:250.

    [Google Scholar]

11.  Allison J (2006) GEANT4 developments and applications. IEEE T Nucl Sci 53:270.

    [Crossref] [Google Scholar]

12. Apostolakis J (2009) Geometry and physics of the GEANT4 toolkit for high and medium energy applications. Radiat Phys Chem 78:859.

    [Crossref] [Google Scholar]

13. Lamrabet A, Maghnouj A, Tajmouati J (2019) Production threshold impact on a GEANT4 calculation of the power deposition in a fast domain: MEGAPIE spallation target. Nucl Sci Technol 30:75.

    [Crossref] [Google Scholar]

14. Lamrabet A, Maghnouj A, Tajmouati J (2019) Assessment of the power deposition on the MEGAPIE spallation target using the GEANT4 toolkit. Nucl Sci Technol 30:54.

    [Crossref] [Google Scholar]

15. Santin G, Strul D, Lazaro D (2003) GATE: A GEANT4-based simulation platform for PET and SPECT integrating movement and time management. IEEE Trans Nucl Sci 50:1516-1521.

    [Crossref] [Google Scholar]

16. Strulab D, Santin G, Lazaro D (2003) GATE (geant4 application for tomographic emission): A PET/SPECT general-purpose simulation platform. Nucl Phys B 125:75-79.

    [Crossref] [Google Scholar]

17. Arce P, Lagares JI, Harkness-Brennan LJ (2014) Gamos: A framework to do Geant4 simulations in different physics fields with an user-friendly interface. Nucl Instrum Methods A 735:304-313.

    [Crossref] [Google Scholar]

18.  Malyshkin Y, Pshenichnov I, Mishustin I (2012) Neutron production and energy deposition in fissile spallation targets studied with GEANT4 toolkit. Nucl Instrum Methods B 289:79-90.

    [Crossref] [Google Scholar]

19.  Malyshkin Y (2012) Modeling spallation reactions in tungsten and uranium targets with the GEANT4 toolkit. EPJ Web Conf 21:10006.

    [Crossref] [Google Scholar]

20. Malyshkin Y, Pshenichnov I, Mishustin I (2014) Monte Carlo modeling of spallation targets containing uranium and americium. Nucl Instrum Methods B 334:8-17.

    [Crossref] [Google Scholar]

21. Mishustin I, Malyshkin Y, Pshenichnov I (2015) Possible production of neutron rich heavy nuclei in fissile spallation targets, in Nuclear Physics: Present and Future. Springer, Cham 151-161.

    [Google Scholar]

22.  Apostolakis J, Asai M, Cosmo G, Howard A, Ivanchenko V, et al. (2008) Parallel geometries in Geant4: Foundation and recent enhancements. IEEE Nucl Sci Symp Conf Rec 883-886.

    [Crossref] [Google Scholar]

23.  Meo SL, Corte´s-Giraldo MA, Massimi C (2015) GEANT4 simulations of the nTOF spallation source and their benchmarking. Eur Phys J A 51:160.

    [Crossref] [Google Scholar]

24. Andersson B, Gustafson G, Nilsson-Almqvist B (1987) A model for low-pT hadronic reactions with generalizations to hadronnucleus and nucleus-nucleus collisions. Nucl Phys B 281:289-309.

    [Crossref] [Google Scholar]

25. Pi H (1992) An event generator for interactions between hadrons and nuclei-FRITIOF version 7.0. Comput Phys Commun 71:173-192.

    [Crossref] [Google Scholar]

26. Quesada JM, Ivanchenko V, Ivanchenko A (2011) Recent developments in pre-equilibrium and de-excitation models in GEANT4. Prog Nucl Sci Technol 2:936-941.

    [Google Scholar]

27. Benlliure J (1998) Calculated nuclide production yields in relativistic collisions of fissile nuclei. Nucl Phys A 628:458-478.

    [Crossref] [Google Scholar]

28.  Gaimard JJ, Schmidt KH (1991) A reexamination of the Abrasion-Ablation model for the description of the nuclear Lowfragmention reaction. Nucl Phys A 531:709-745.

    [Crossref] [Google Scholar]

29. Junghans AR (1998) Projectilefragment yields as a probe for the collective enhancement in the nuclear level density. Nucl Phys A 629:635-655.

    [Crossref] [Google Scholar]

30. Garcı´a AR, Mendoza E, Cano-Ott D (2013) Validation of the thermal neutron physics in GEANT4, Department of Energy, Madrid.

    [Google Scholar]

31. Hartling K, Ciungu B, Li G (2018) The effects of nuclear data library processing on Geant4 and MCNP simulations of the thermal neutron scattering law, Nuclear Inst. Methods Phys Res A 891:25-31.

    [Crossref] [Google Scholar]

32. Li G, Bentoumi G, Tun Z (2018) Application of GEANT4 to the data analysis of thermal neutron scattering experiments. CNL Nucl Rev 7:11-17.

    [Crossref] [Google Scholar]

33. Li G, Bentoumi G, Hartling K (2020) Validation of ENDF/B-VIII thermal neutron scattering data of heavy water by differential cross section measurements at various temperatures. Ann Nucl Energy 135:106932.

    [Crossref]

34. Lamrabet A, Maghnouj A, Tajmouati, J (2021) GEANT4 characterization of the neutronic behavior of the active zone of the MEGAPIE spallation target. Nucl Eng Technol 53:3164-3170.

    [Crossref] [Google Scholar]